Climate🔗

Introduction🔗

Like the carbon cycle, the climate sector is adapted from the FREE model, which used the DICE climate sector without modification (Nordhaus 1994). The DICE structure in turn followed Schneider and Thompson (1981).

The model has been recast in terms of stocks and flows of heat, rather than temperature, to make the physical process of accumulation clearer to users. However, the current model is analytically equivalent to the FREE and DICE versions. While FREE and DICE used exogenous trajectories for all non-CO₂ radiative forcings, this version adds endogenous forcings from all well-mixed GHGs, i.e., CO₂, CH₄, N₂O, PFCs, SF₆, and each HFC type.

Structure🔗

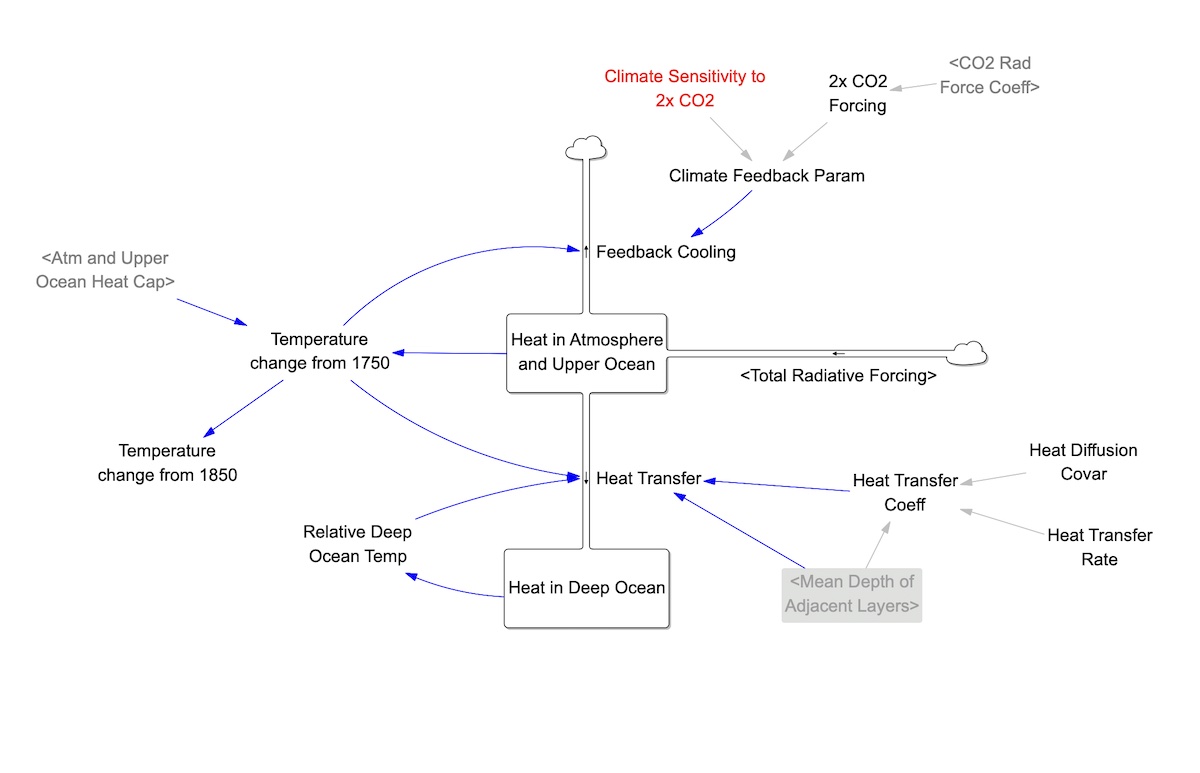
The model climate is a fifth-order, linear system, with three negative feedback loops. Two loops govern the transport of heat from the atmosphere and surface ocean, while the third represents warming of the deep ocean. Deep ocean warming is a slow process, because the ocean has such a large heat capacity. If the deep ocean temperature is held constant, the response of the atmosphere and surface ocean to warming is first-order. Temperature change is a function of radiative forcing (RF) from greenhouse gases and other factors, feedback cooling from outbound longwave radiation, and heat transfer from the atmosphere and surface ocean to the deep ocean layer.

$$ \begin{align} T_\mathrm{surf} &= \frac{Q_\mathrm{surf}}{R_\mathrm{surf}} \\[4pt] T_\mathrm{deep} &= \frac{Q_\mathrm{deep}}{R_\mathrm{deep}} \\[4pt] Q_\mathrm{surf} &= \int (RF(t) - F_\mathrm{out}(t) - F_\mathrm{deep}(t))\,dt + Q_\mathrm{surf}(0) \\[4pt] Q_\mathrm{deep} &= \int F_\mathrm{deep}(t)\,dt + Q_\mathrm{deep}(0) \end{align} $$

T = temperature of surface and deep ocean boxes
Q = heat content of respective boxes
R = heat capacity of respective boxes
RF = radiative forcing
F_out = outgoing radiative flux
F_deep = heat flux to deep ocean

$$ \begin{align} F_\mathrm{out}(t) &= \lambda \, T_\mathrm{surf} \\[4pt] F_\mathrm{deep}(t) &= R_\mathrm{deep} \cdot \frac{T_\mathrm{surf} - T_\mathrm{deep}}{\tau} \end{align} $$

λ = climate feedback parameter
τ = heat transfer time constant

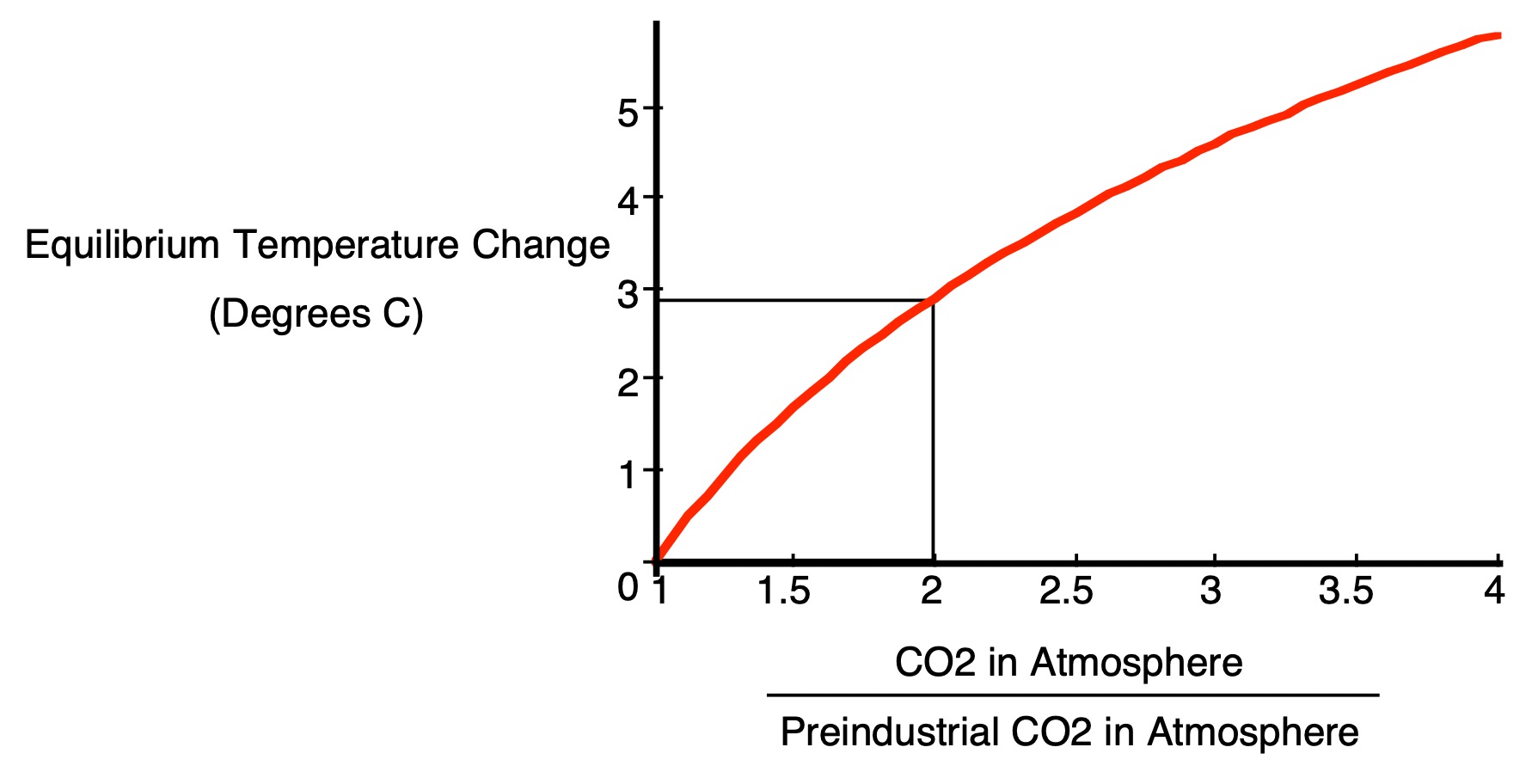
Radiative forcing from CO₂ is logarithmic of the atmospheric CO₂ concentration (IPCC AR6, 2023; NOAA, 2024). Forcing from CH₄ and N₂O is less than the sum of RF from each individually to account for interactions between both gases. Forcing from each F-gas is the product of its concentration and its radiative forcing coefficient; the total forcings of F-gases is the sum of these products, as are the forcings from MP gases derived. The sum of other forcings, which include those from aerosols (black carbon, organic carbon, sulfates), tropospheric ozone, defaults to an exogenous time-varying parameter. The values use a composite of AR6 history 1750-2019 and their projections for SSP4 6.0 through 2100. The equilibrium temperature response to a change in radiative forcing is determined by the radiative forcing coefficient, κ, and the climate feedback parameter, λ. Equilibrium sensitivity to 2xCO₂eq forcing is 3°C in the base case.

$$ T_\mathrm{equil} = \frac{\kappa \cdot ln \bigl( \frac{C_a}{C_\mathrm{a,0}} \bigr)}{\lambda \cdot ln(2)} $$

T_equil = equilibrium temperature
C_a = atmospheric CO₂ concentration
C_a,0 = preindustrial atmospheric CO₂ concentration
κ = radiative forcing coefficient
λ = climate feedback parameter

Model Structure🔗